Lesson 8 – Capitalization: Converting an Income Stream into Value (The Income Approach to Value)

Capitalization is any method used to convert an income stream into value. There are two primary income capitalization methods: direct capitalization and yield capitalization. (A capitalization rate is any rate used to convert an estimate of future income into an estimate of market value.

Direct Capitalization is a method used to convert an estimate of a single year's income expectancy into an indication of value in one direct step. Dividing the income estimate by an appropriate rate or by multiplying the income estimate by an appropriate factor converts the income stream into an estimate of value. In essence, direct capitalization expresses value as a relationship between income and a rate or multiplier. The direct capitalization technique employs capitalization rates and multipliers extracted from comparable sales. Yield and value changes are implied, but not directly identified.

How to derive value by direct capitalization will be discussed in detail in Lesson 9 (Multipliers – Derivation and Valuation) and Lesson 12 (Valuation of Property using Overall Rates).

Yield Capitalization is a capitalization method used to convert future benefits into present value by discounting each future benefit at an appropriate yield rate. The future benefits may also be discounted by developing an overall capitalization rate that explicitly reflects the investment's income pattern, value change, and yield rate. As such, this method is also known as the discounted cash flow (DCF) model. The yield rate represents the multi period rate of return that an investor would expect when investing in the property given the risk of the income stream. Yield capitalization explicitly considers the size, shape, and duration of the income stream and any change in the value of the property. Future income is discounted using the present value factors (PW1 and PW1/P).

How to derive yield capitalization rates will be discussed in Lesson 13 (Derivation of Yield Rates).

1. Appraisal Institute, The Dictionary of Real Estate Appraisal, Fourth Edition, page 83.

Generally, income streams are converted into indicators of value by using rates and factors. The two basic formulas are:

• Income divided by a rate equals value: I ÷ R = V.
• Income multiplied by a factor (multiplier) equals value: I × F = V = I × M.

The key variables of income capitalization include: (1) the income to be capitalized; (2) the capitalization rate or factor used to convert the income into a value indicator; and (3) the time period over which the income is to be realized. The capitalization rate or factor must provide for both the return OF the portion of the investment and the return ON the investment.

Formula 1 – Income Divided by a Rate Equals Value

In its simplest form, the capitalization process may be represented by the equation:

V = I ÷ R

Where:

From the basic formula of V = I ÷ R, we can (algebraically) derive that:

I = R × V

From the basic formula of V = I ÷ R, we can also (algebraically) derive that:

R = I ÷ V

This can also be summarized in the following "T" graph, a mnemonic device that some find useful – think of it as "IRV":

To use IRV, the horizontal line, “—”, represents a division line, and the vertical line, "|", represents multiplication. Therefore, if you were trying to solve for "R," you would merely cover the "R" with your finger and use the remaining formula visible (I ÷ V) to solve for "R." Likewise, if you were trying to solve for "I," you would cover the "I" with your finger and use the remaining formula visible (R × V) to solve for "I." Lastly, if you were solving for "V," you would cover the "V" with your finger and the remaining formula would be I ÷ R.

Demonstration of Income Divided by a Rate Equals Value Formula

DEMONSTRATION 8-1: Solving for Value

In this demonstration, we will show you how to solve for value using the basic formula V = I ÷ R (value equals income divided by rate) which was illustrated in Example 8-1 of this lesson.

A property has operating expenses that are 32 percent of the gross income; the gross income is $125,000. Using a 14 percent capitalization rate, what is the value of the property?

SOLUTION: Value = Income ÷ Rate.

Income = $125,000 – ($125,000 × 0.32) = $85,000

Value = $85,000 ÷ 0.14 = $607,143.

DEMONSTRATION 8-2: Solving for Income

In this demonstration, we will show you how to solve for value using the basic formula V = I ÷ R (value equals income divided by rate) which was illustrated in Example 8-2 of this lesson. As discussed from the basic formula, we can algebraically derive other equations, such as I = R × V or R = I ÷ V. If you are solving for income, as we are doing in this demonstration, you must use the equation I = R × V (income equals rate multiplied by value.)

You bought an investment property for $225,000. You estimate a resale price of $300,000. If the new buyer requires a 9 percent rate of return, what is the projected net operating income?

SOLUTION: Income = Rate × Value.

Income = .09 × $300,000 = $27,000.

Only the current selling price is relevant to the current net operating income.

DEMONSTRATION 8-3: Solving for Rate

In this demonstration, we will show you how to solve for value using the basic formula V = I ÷ R (value equals income divided by rate) which was illustrated in Example 8-3 of this lesson. As discussed from the basic formula, we can algebraically derive other equations, such as I = R × V or R = I ÷ V. If you are solving for rate, as we are doing in this demonstration, you must use the equation R = I ÷ V (rate equals income divided by value.)

A property has a NOI (net operating income) of $15,000, debt service of $10,000, and sold for $100,000. What is the overall capitalization rate?

SOLUTION: Rate = Income ÷ Value.

Rate = $15,000 ÷ $100,000 = 15 percent

The debt service does not enter into the calculation of an overall capitalization rate.

Note: Incidentally, this calculated rate, 15 percent in this instance, is called the OverAll Rate [OAR]. It will be discussed in detail starting in Lesson 11: Derivation of Overall Rates [OARs] from Sales and by Band of Investment.

Formula 2 – Income Multiplied by a Factor (Multiplier) Equals Value

A single year's gross income may be converted to an indicator of value by multiplying the income by an income multiplier derived from the sales of comparable properties. This method is mathematically related to direct capitalization since a capitalization rate is the reciprocal of an income multiplier or factor (although an income multiplier is generally based on a gross level of income, while an overall capitalization rate is based on a net level of income). The multiplier or factor process may be represented by the equations:

V = I × M

Where:

Replacing M with F, where F = the factor

V = I × F

From these formulae (interchangeably using M and F), we can (algebraically) derive that:

M = V ÷ I

From the basic formula of M = V ÷ I, we can (algebraically) derive that:

I = V ÷ M

Again, this can also be summarized in the following "T" graph, "VIM":

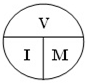
To use VIM, the horizontal line, “—,” represents a division line, and the vertical line, "|," represents multiplication. Therefore, if you are trying to solve for "M," you would merely cover the "M" with your finger and use the remaining formula visible is (V ÷ I) to solve for "M." Likewise, if you were trying to solve for "I," you would cover the "I" with your finger and use the remaining formula (V ÷ M) to solve for "I." Lastly, if you were solving for ";V," you would cover the "V" with your finger and the remaining formula would be I × M.

Demonstration of Income Multiplied by a Factor Equals Value Formula

DEMONSTRATION 8-4: Solving for Value

In this demonstration, we will show you how to convert an income stream into value using the formula of V = I × M (value equals income multiplied by multiplier) which was illustrated in Example 8-4 of this lesson.

A warehouse rent for $1,500 a month. The gross income multiplier is 11. What will the warehouse sell for on the open market?

SOLUTION: Value = Income × Multiplier

Income = $1,500 × 12 months = $18,000 per year.

Value = ($1,500 × 12) × 11 = $198,000

DEMONSTRATION 8-5: Solving for Multiplier

In this demonstration, we will show you how to solve for a multiplier using the basic formula of V = I × M (value equals income multiplied by multiplier) which was illustrated in Example 8-5 of this lesson. As discussed from the basic formula, we can algebraically derive other equations, such as M = V ÷ I or I = V ÷ M. If you are solving for a multiplier, which we are doing in this demonstration, you must use the equation M = V ÷ I (multiplier equals value divided by income.)

Given a sale of $750,000 and gross income of $66,000, what is the indicated gross rent multiplier?

SOLUTION: Multiplier = Value ÷ Income.

Multiplier = $750,000 / $66,000 = 11.36

DEMONSTRATION 8-6: Solving for Income – using multipliers

In this demonstration, we will show you how to solve for a multiplier using the basic formula of V = I × M (value equals income multiplied by multiplier) which was illustrated in Example 8-6 of this lesson. As discussed from the basic formula, we can algebraically derive other equations, such as M = V ÷ I or I = V ÷ M. If you are solving for a income, which we are doing in this demonstration, you must use the equation I = V ÷ M (income equals value divided by multiplier.)

A 10-unit apartment building is offered for sale at $990,000. Comparable properties indicate a gross rent multiplier of 8.25. What is the annual rent per unit?

SOLUTION: Income = Value ÷ Multiplier.

Income = $990,000 ÷ 8.25 = $120,000 ÷ 10 units = $12,000

Rates vs. Factors (Multipliers)

Rates and factors are mathematical reciprocals of each other. A reciprocal is the quantity resulting from the dividing one by a given number. For example, an income of $100 multiplied by a factor of 20 produces a valuation of $2,000. The reciprocal of 20 (1 divided by 20) is 0.05. Dividing $100 by the rate of 0.05 also produces a valuation of $2,000.

If an income stream is converted into a value estimate by multiplying the income times a number, that number is a factor. If an income stream is converted into a value estimate by dividing the income by a number, then that number is a rate.

The lesson you just read discussed the two primary methods of converting an income stream into value: the direct capitalization method and the yield capitalization method. It also explained that the two basic formulas used to convert income streams to an indicator of value:

I ÷ R = V (Income divided by Rate equals Value) and I × F = V (Income multiplied by Factor – commonly referred to as a multiplier – equals Value).

The next lesson, Lesson 9, (Multipliers – Derivation and Valuation), will explain how to derive multipliers and how to value by direct capitalization using multipliers.

M = V ÷ I : GIM = SP ÷ Ant GI and Eff GIM = SP ÷ Ant Eff GI

Anticipated Gross Income, or Anticipated Effective Gross Income, is used in deriving multipliers.

V = I × M : V = PGI × GIM and V = Eff GI × Eff GIM

Market – economic – income is used in valuing property.

Lesson 10 provides a further review of multipliers, rates, and factors.

Then, in Lesson 11, we will discuss the derivation of OverAll Rates, either by derivation using the Band of Investment method, or by extracting rates from sales of comparable properties:

R = I ÷ V : OAR = Ant NIBR ÷ SP or OAR = Ant NOI ÷ SP … remember, Net Operating Income is the same as the Net Income Before deducting for Recapture, after an allowance for Vacancy and Collection Losses is made and after all Operating Expenses – fixed (including Property Taxes), variable, and reserves for replacement – have been deducted.

Remember also – anticipated NIBR (or NOI) is used in extracting a rate from a sale.

This will be followed by how to derive value by direct capitalization, in detail, in Lesson 12 (Valuation of Property using Overall Rates).

V = I ÷ R

We will learn in Lesson 12 that, when valuing property for ad valorem property tax purposes, the income to be capitalized, using an OverAll Rate, is the Net Income Before deducting for recapture and property Taxes, NIBT. Moreover, the capitalization rate will include both the OverAll Rate and the Effective property Tax Rate.

Yield capitalization will be discussed beginning in Lesson 13, where we will discuss the derivation of straight-line declining terminal yield rates and level terminal yield rate.

Lesson 14 will examine the different income streams introduced in Lesson 6, and match these income streams with the different methods of capitalization. Subsequent lessons will delve further into these methods, and some of the basic techniques the appraiser uses to value property using the income approach to value.